Making Cross-Correlated EVM Measurements
Cross-Correlated EVM Overview
Cross-Correlated EVM Error vector magnitude (EVM): A quality metric in digital communication systems. See the EVM metric in the Error Summary Table topic in each demodulator for more information on how EVM is calculated for that modulation format. (ccEVM) computes EVM by combining the results of two different measurements of the same signal so as to reduce the noise contributed by the acquisition hardware. This procedure causes the ccEVM value to primarily contain the noise coming from the DUT Device under Test: An acronym used to describe some type of electrical apparatus connected to test instrumentation. The apparatus can range from a single component to a complex subsystem such as a mobile phone, base station or MSC. (or source and DUT, in the case of an amplifier).
Cross-Correlated EVM is only available when the number of input channels is greater than one, and even (2, 4, 6, etc.). Two measurements will be performed on half of the measurement channels. Each measurement will be assigned a contiguous block of channels (channels 1 to N/2 assigned to Meas1, channels N/2+1 to N assigned to Meas2).
Cross-Correlated EVM is enabled in the measurement extension's Demod Properties dialog, and CcEVM results, Valid Points and Total Points are displayed in the Cross-Correlated EVM Summary trace. Refer to the measurement extension's help topics for more measurement-specific information.
MIMO CcEVM Configuration Examples
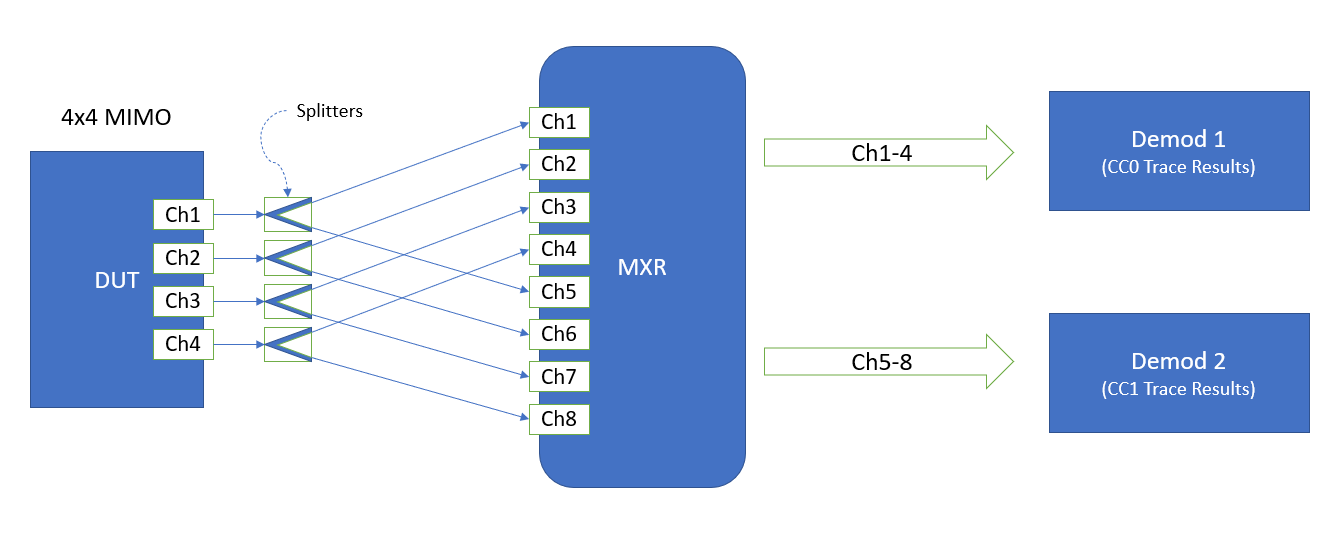
Some measurement extensions (e.g., 5G NR, Custom OFDM and 802.11n/ac/ax/be) support MIMO Multiple Input, Multiple Output: A physical layer (PHY) configuration in which both transmitter and receiver use multiple antennas. Cross-Correlated EVM measurements. Two example MIMO CcEVM configurations are shown below.
Example 2x2 MIMO CcEVM Configuration

Example 4x4 MIMO CcEVM Configuration
See Also
Cross-Correlated EVM (Custom OFDM)
